Publications

In this paper we present a unified treatment of three-body atoms and molecules using numerical tensor methods. The Schrödinger equation in perimetric coordinates is recast in a canonical tensor format. It is shown that the Schrödinger equation can be solved in this full-tensor format but that by using a low-rank tensor decomposition, in particular the tensor-train and quantized-tensor-train formats, energies accurate to at least the nanohartree can be obtained for the He atom (in which the mass of the uniquely charged particle is much greater than the other two particles), the positronium negative ion (Ps${}^{−}$, in which all the masses are equal), and the non-Born-Oppenheimer H${}_2⁢^{+}$ molecule (in which the mass of the uniquely charged particle is much smaller than the other two particles).

K. T. Brice, M. Melgaard, H. Cox

Physical Review A 609 (2024), 062812.

We provide a mathematical justification of a spectral approximation scheme known as spectral binning for the Kohn–Sham spin density-functional theory in the presence of an external (nonuniform) magnetic field and a collinear exchange-correlation energy term. We use an extended density-only formulation for modeling the magnetic system. No current densities enter the description in this formulation, but the particle density is split into different spin components. By restricting the exchange-correlation energy functional to be of a collinear LSDA form, we prove a series of results which enable us to mathematically justify the spectral binning scheme using the method of Gamma-convergence, in conjunction with auxiliary steps involving recasting the electrostatic potentials, justifying the spectral approximation by making a spectral decomposition of the Hamiltonian and linearizing the latter Hamiltonian.

M. Melgaard, V. J. J. Syrjanan

Journal of Mathematical Chemistry 62 (2024), 711–760.

We consider the positive solutions of some integral systems related to the static Hartree type equations. Firstly, assuming that the exponent p, see paper abstract for details, belongs to some suitable interval depending the parameters mu, tau, alpha, and beta, we are able to prove some nonexistence results for the positive solution. Secondly, we also establish some qualitative results for the integrable solution of the system like regularity, symmetry and asymptotic behaviour. As a corollary, we deduce the corresponding results for the equivalent weighted Hartree type nonlocal equations. The results obtained in this paper generalize and complement the existing results in the literature.

M. Melgaard, M. Yang, Minbo, X. Zhou

Pacific Journal of Mathematics 317 (2022), no. 1, 153-186

We study the nonlinear, nonlocal, time-dependent partial differential equation $i \pd_{t}\vf=(\sqrt{ -\D +m^{2} } -m) \vf -\left( \frac{1}{|x|} \ast | \vf |^{2} \right)\vf$ on $\R^{3}$, which is known to describe the dynamics of quasi-relativistic boson stars in the mean-field limit. For positive mass parameter $m>0$ we establish existence of infinitely many (corresponding to distinct energies $\l_{k}$) travelling solitary waves, $\vf_{k}(x,t) = e^{i \l_{k} t } \f_{k}(x-vt)$, with speed $|v| <1$, where $c=1$ corresponds to the speed of light in our choice of units. These travelling solitary waves cannot be obtained by applying a Lorentz boost to a solitary wave at rest (with $v=0$) because Lorentz covariance fails. Instead we study a suitable variational problem for which the functions $\f_{k} \in \Hb^{1/2}(\R^{3})$ arise as solutions (called boosted excited states) to a Choquard type equation in $\R^{3}$, where the negative Laplacian is replaced by the pseudo-differential operator $\sqrt{ -\D +m^{2} } -m$ and an additional term $i (v \cdot \nabla)$ enters. Moreover, we give a new proof for existence of boosted ground states. The results are based on perturbation methods in critical point theory.

M. Melgaard, F. Y. Zongo

Analysis and Applications 20 (2022), no. 2, 285-302

We study the self-adjoint Dirac operators $D=\ham{D}_0 + V(x)$, where $D_0$ is the free three-dimensional Dirac operator and $V(x)$ is a smooth compactly supported Hermitian matrix potential. We define resonances of $D$ as poles of the meromorphic continuation of its cut-off resolvent. By analyzing the resolvent behaviour at the spectrum edges $\pm m$, we establish a generalized Birman-Krein formula, taking into account possible resonances at $\pm m$. As an application of the new Birman-Krein formula we establish the Poisson wave trace formula in its full generality. The Poisson wave trace formula links the resonances with the trace of the difference of the wave groups. The Poisson wave trace formula, in conjunction with asymptotics of the scattering phase, allows us to prove that, under certain natural assumptions on $V$, the perturbed Dirac operator has infinitely many resonances; a result similar in nature to Melrose’s classic 1995 result for Schrödinger operators.

B. Cheng, M. Melgaard

Asian Journal of Mathematics 25 (2021), no. 2, 243-276

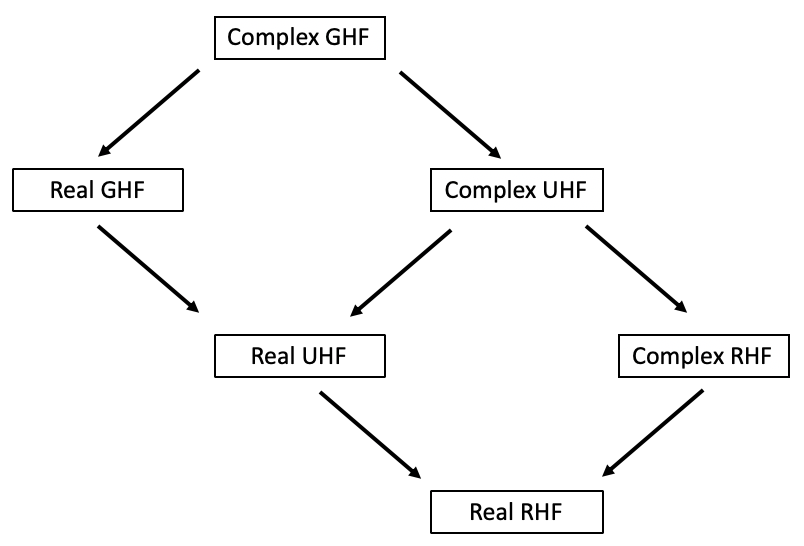
This review brings together mathematical proofs and high-accuracy quantum chemical calculations on the hydride ion, the anion of hydrogen. Our discussion is confined to Hartree-Fock theory and the non-relativistic time-independent Schroedinger equation, within the fixed-nucleus approximation. It is written so as to be accessible to both the mathematics and physical chemistry communities.

H. Cox, A. L. Baskerville, V. J .J. Syrjanen, M. Melgaard

Advances in Quantum Chemistry 81 (2020), 167-189

Within the Hartree-Fock theory of atoms and molecules we prove existence of a ground state in the presence of an external magnetic field when:(1) the diamagnetic effect is taken into account; (2) both the diamagnetic effect and the Zeeman effect are taken into account. For both cases the ground state exists provided the total charge $Z_{\rm tot}$ of the nuclei $K$ exceeds $N-1$, where $N$ is the number of electrons. For the first case, the Schrödinger case, we complement prior results by Esteban-Lions (1989) and Enstedt-Melgaard (2008) by allowing a wide class of magnetic potentials. In the second case, the Pauli case, we include the magnetic field energy in order to obtain a stable problem and we assume $Z_{\rm tot} \a^{2} \leq 0.041$, where $\a$ is the fine structure constant.the diamagnetic.

C. Argaez, M. Melgaard

Applicable Analysis 97 (2018), no. 14, 2377-2403